Bioeconomics
| A Wikibookian believes this page should be split into smaller pages with a narrower subtopic. You can help by splitting this big page into smaller ones. Please make sure to follow the naming policy. Dividing books into smaller sections can provide more focus and allow each one to do one thing well, which benefits everyone. |
| A reader requests expansion of this book to include more material. You can help by adding new material (learn how) or ask for assistance in the reading room. |
Bioeconomics is the theory of economic exploitation of living resources, dealing with two dynamic systems: population dynamics and the dynamics of economic systems. Bioeconomics therefore leans on two traditional university disciplines, biology and economics.
Short reference to production theory[edit | edit source]
Production theory is a central element in microeconomics and describes simply the conversion of inputs (v) into outputs (Q):
|
There are several ways of specifying this function. One is as an additive production function:
|
where p0, p1, .... pn are parameters that are determined empirically.
Another is as a Cobb-Douglas production function (multiplicative):
|
Other forms include the constant elasticity of substitution production function (CES) which is a more general formulation including the Cobb-Douglas function, and the quadratic production function which is a specific type of additive function. The best form of the equation to use and the values of the parameters vary from company to company and industry to industry.
In a short run production function at least one of the (inputs) is fixed. In the long run all factor inputs are variable, in principle at the discretion of management.
In classical theory production may involve three types of input: Labour (L), Capital (K) and Natural resources (R). Some classical works splits the latter into two: Natural resources and Energy resources (E). More often outputs from other production processes are used as inputs others. But in principle it should be possible to separate all inputs down to the three or four basic types of input.
Catch production in the short run[edit | edit source]
The simplest model of catch production involves only two input factor: A natural resource (x) and a fishing activity (fishing effort, F).
Production of fishing effort[edit | edit source]
Let us start to discuss the production of a certain quantity of fishing effort. Further let us assume that human labour input (which could be regarded as a natural resource, but is more practically described as labour) and Capital (boats, fishing gears, etc.) is the two basic types of input in the production of fishing effort. Also assume one of the two factors could be perfectly substituted by the other in a certain quantity.
|
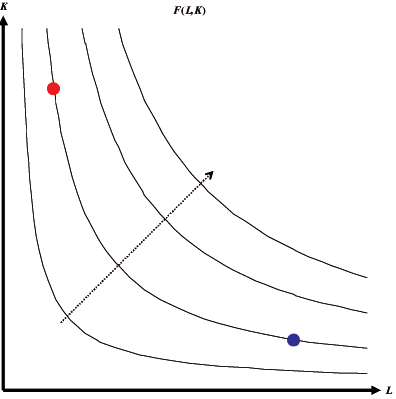
While limiting the input factor to two, the substitution rates can easily be viewed in a contour plot, often referred to as isocurves of production (Fig. 3.1).
| Figure 3.1 Isocurves of fishing effort production. The curves show at which rate L (Labour) can be substituted by K (Capital) and the other way around, each curve representing a constant output of F. The blue point is representing a certain production of fishing effort involving a high degree of labour inputs, while much of the labour in the red point is substituted by capital, e.g. small boats and hand line (blue point) vs. a few trawlers (red point). The dotted arrow indicates increase in effort production. |
The concept of efficient production[edit | edit source]
Equation 3.1 is simply giving a technical description on how F is produced by the input factor L and K and does not give us any suggestions on which mix of input factors are to be preferred. In order to prioritize between different alternatives of producing a specific quantity of F, it is convenient to look at the cost of the two input factors. Let the unit cost of labour (L) be w and the unit cost of capital (K) be r. The total cost of production (C) then is:
|
Lagrange's method can be used in order to maximise the production of fishing effort at a cost constraint, which is the dual problem of minimising the cost at a given production. The Lagrange equation will be:
|
where C0 is the given cost and the Lagrange multiplier.
The first order condition when maximising the Lagrange equation, is that the partial derivatives of the equation with respect of L and K equals 0, from which follows:
|
This expression is referred to as the marginal rate of technical substitution (MRTS). In the most cost-efficient production MRTS should according to Eq. (3.4) equal the price ratio of the two input factors. Cost efficient solutions of different levels of production are shown in Figure 3.2.
| Figure 3.2 Efficient production. The thick line connects the infinite number of points consistent with equation (3.4), when varying the cost constraint C0 |
Producing fish harvest by two input factors[edit | edit source]
By regarding production of fishing effort as an independent production process, production of (h, fish harvest) can be expressed by the two input factors x and F:
|
It is reasonable to assume that x and F is substitutable in the same way as L and K in Figure 3.1. In order to fish a certain quantity, say one kilo fish, when the stock biomass is low (low x-value), one has to input a larger fishing effort than in the case of a higher stock density (large x-value).
Eq. (3.5) therefore is of the same type as Eq. (3.1) and in principle we have the same type of continuous substitution as indicated in Figure 3.1.
Market failures while using common resources[edit | edit source]
There are however two core issues which turns this production into a drastically different task:
|
The first problem makes it impossible to continue along the normal methods of identifying cost efficient solutions. Since the scarcity of the natural resource is not reflected in a price, we lack the value information on this factor. The other problem is also corrupting our model, as it attacks the basic assumption of independency in availabilities of the two input factors. In the long run in fact we have:
|
The straight forward implication of this is obvious from Eq. (3.5):
|
showing that in the long run (keeping fishing effort constant over a sufficient period of time) catch will be determined by the fishing effort alone.
The crucial relationship to investigate further therefore is the x-F relationship. How the stock biomass x be defined as a function of fishing effort F? At this point we have to turn to biology and population dynamics.
Population growth[edit | edit source]
Population dynamics is the study of marginal and long term changes in numbers, individual weights and age composition of individuals in one or several populations, and biological and environmental processes influence those changes. In idealized population growth models one differs between compensatory growth and decompensatory growth, the first one is regarded as normal growth.
The logistic growth model is a widely-used compensatory growth model.
Logistic growth[edit | edit source]
Let us assume annual biomass growth of a fish population to follow logistic growth (first proposed as a demographic model by Verhulst, 1838. (Applied as a biomass growth model by Pearl, 1934.) The population dynamics is described by a differential equation where biomass (x) is a function of time (t) and the time derivative of population biomass is:
|
Note that the two parameters (constants), r often referred to as the intrinsic growth rate and K the population biomass at natural equilibrium, are not the same as the parameter r and the variable K above (in pnt. 3).
The parabolic function (square function) in Eq. 4.1, shown in Figure 4.1, describe and increasing biomass growth as the population biomass increases, up to a certain population size (which is easy to identify as K/2), where the biomass growth starts declining to reach zero at biomass level K. K therefore represents a natural equilibrium biomass of an unexploited stock.
| Figure 4.1 Equation 4.1, where the time derivative of x is measured along the y-axis |
Biomass growth as a function of time[edit | edit source]
The differential equation (4.1) has a unique solution ( = INTEGRAL):
|
x0 being the biomass at t=0.
Catch production in the long run[edit | edit source]
Let us start with catch production in the short run as discussed above. Eq. (3.5) defines catch as an output from of production process where stock biomass, x, and fishing effort, F, are input factors. x can be substituted by F. This assumption of substitution is taken care of in the so-called Schaefer production equation:
|
q is a constant (parameter) often referred to as the catchability coefficient. By referring to Eq. (2.3) we see that the Schaeffer production equation is of the Cobb-Douglas type, with powers set equal to 1. Later we will investigate the consequences of the choice of power values.
The biomass growth equation (4.1) now has to be adjusted to include catch. The annual biomass growth will be the natural growth (right hand side of 4.1) minus the harvested biomass (e.g. 5.1):
|
As Eq. (4.1) identify K as the natural biomass equilibrium when , Eq. (5.2) also identify an equilibrium biomass when keeping F constant over an infinite number of years. The equilibrium is defined by
|
from which follows (in the case of Eq. 5.2):
|
Skipping the trivial solution x=0, the stock biomass - fishing effort relationship is given directly from Eq. (5.4):
|
The long term catch equation is finally found by inserting Eq. (5.5) into the short term catch equation defined by Eq. (5.1):
|
Catch Revenue and Cost[edit | edit source]
|
|